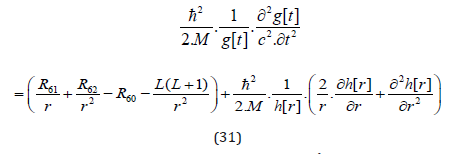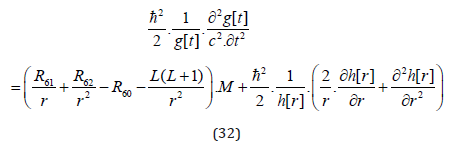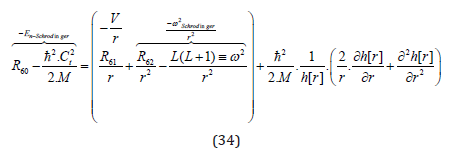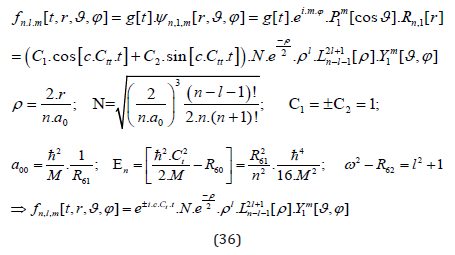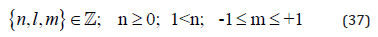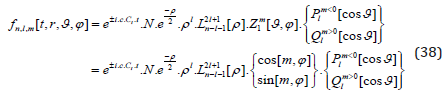In order to keep this paper as brief as possible we only introduce
the essentials. These essentials are a set of new solutions to the
Einstein-Field-Equations as given in [2-34]. Among these solutions,
we also found candidates with the potential to construct Turing or
Turing-like machines [35] having special bits apparently even more
powerful than quantum bits.
In [34] we gave a time dependent (oscillating) solution, which
represented a metric satisfying the Einstein-Field-Equations
[36,37] for the case of an arbitrary 2-EQ-bit (EQ=Einstein Quantum)
processor. Here we intend to investigate these solutions with
respect to possible extensions regarding more bits plus timidly
start to explore the question of storage and machine design in
connection with the quantum property named spin.
As the introduction to the method of quantizing metric solutions
of the Einstein-Field-Equations is quite lengthy and was already
presented in a variety of previous papers [2-34], we refrain from
presenting it here again. Instead, we will only give a brief recipe:
1. We consider space as an ensemble of properties.
2. These properties could just be degrees of freedom and
thus, dimensions, which are subjected to a Hamilton extremal
principle.
3. This leads to the Einstein-Hilbert-Action [36] and
subsequently to the Einstein-Field-Equations [36,37].
4. Time seems to take a special place among the properties
as it is not such a property itself but consists of all other
properties’ internal changes and variations. Applying the
Einstein-Field-Equations on the internal degrees of freedom
of each single property as a one-dimensional space (c.f. [14]),
gives exactly 6 solutions [17] among which we always also
find oscillations. These internal periodic processes (changes)
inside each property or dimension are realized as time from an
external observer… time, which itself forces other properties to
change. Thus, starting as an internal property (solution) within
each dimension, time not only is change, but also brings change
about. Apparently, time is the most fractal and self-similar thing
there is in this universe.
5. Now, however, we have the interesting situation that
solutions to the Einstein-Field-Equations are not necessarily
unique. Derived from the Einstein-Hilbert-Action as an extremal
principle, the starting quantity is the so-called Ricci scalar R,
being the essential kernel of this action. This results in a metric
solution to the Einstein-Field-Equations, being subsequently
derived from the Einstein-Hilbert-Action. Most interestingly,
there are also infinitely many solutions to just one given Ricci
kernel. Just as an example, one might take the flat Minkowski
space and the Schwarzschild vacuum metric [38]. Both have a
vanishing Ricci scalar R=0, but while the first describes empty
space, the second, even though being a vacuum solution,
contains a gravitational “object” of spherical symmetry. And
yes, this all comes out from a variational kernel of R=0. Thus, so
our conclusion, there seem to be quite some degrees of freedom
regarding the choice of metric solutions to just one Ricci scalar
curvature.
6. Tickling metric solutions with respect to this degree
of freedom, which is to say to perform a variation (or
transformation) of metric solutions, thereby treating the Ricci
scalar as a conserved quantity, gives us classical quantum
equations and thus Quantum Theory.
Essentially one finds Quantum Gravity as transformations to
metrics solving the Einstein-Field-Equations:

Here we have: R
αβ,T
αβ the Ricci- and the energy momentum
tensor, respectively, while the parameters Λ and κ are constants
(usually called cosmological and coupling constant, respectively).
These are the well-known Einstein-Field-Equations in n dimensions
with the indices α and β running from 0 to n-1. The theory behind is
called “General Theory of Relativity”.
Thereby we can use external or internal degrees of freedom.
While in most of our previous papers (e.g. [33] as this is
most compact) we concentrated on external or wrapper-like
transformations of the kind:
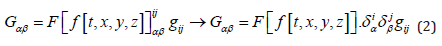
we also introduced inner or Killing-like approaches in [39].
Most interestingly, these inner Quantum Gravity transformations
led to the Dirac equation [40].
In conclusion we might state that Quantum Theory is just the
inner degree or fluctuation of metric solutions to the Einstein-
Field-Equations.
The interested reader will find a compact mathematical
presentation of the above recipe in [33].
It is a common misinterpretation of the original Turing work
[35] that many people assume Turing has suggested a digital
machine. In fact, his approach contained a computer machine with
an arbitrary real basis, but Turing considered the use of integer
numbers to be the most appropriate way to realize his machine and
so he suggested the digital form. In principle, however, his concept
was not necessarily restricted to a digital basis.
As we found that the Einstein-Field-Equations are the most
principle building blocks of this universe, we want to find solutions
to these equations, which – at least in theory – allow us to build a
computer. It is obvious that such a system requires a circuit time,
governing the whole system and two additional properties allowing
for storage and operations.
The corresponding evaluation was already performed
elsewhere [2,34,41]. For convenience we here repeat it briefly.
By applying a metric approach of the kind:
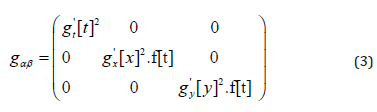
we will find a general solution to the Einstein-Field-Equations
[1, 3] with the function:

Thereby Λ denotes the cosmological constant. Please note the
matter and anti-matter character of our solution (4). The functions
g
x and g
y are arbitrary and could be used for operations/data of any
arbitrary form. In order to assure digital outcomes to the metric
components g
11 and g
22, standing for the second and the third
diagonal metric component, respectively, we construct Fourier
series of the kind:
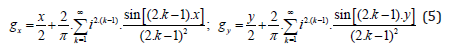
We see that the system constructed above has everything a most
simple 2-bit “processor” requires. It has the necessary two bits and
it has a periodic function defining a cycle time. What we also need is
a way to store information. Applying the Turing approach [35], we
might just couple in certain add-on dimensions playing the role of
these storages, or, if using the Turing picture, playing the role of the
tape of our EQ-Turing machine (EQ=Einstein Quantum). A simple
extension of the metric (3) of the form:
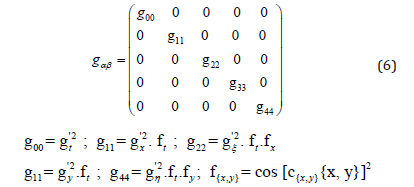
might help us to solve the problem for at least one storage
process by entangling the coordinates x-ξ and x-η. A second set of solutions can be constructed via the following approach:
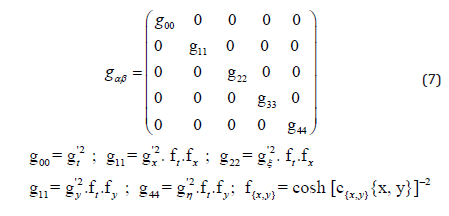
and would bring the same result in a slightly variated form
(read and write options).
We recognize the application of the pairwise coupling or
entanglement of dimensions as elaborated in [42]. Substituting
ξ=xx and η=yy we can directly obtain the solutions from [34] with
the following sub-metric of the components 1-4:
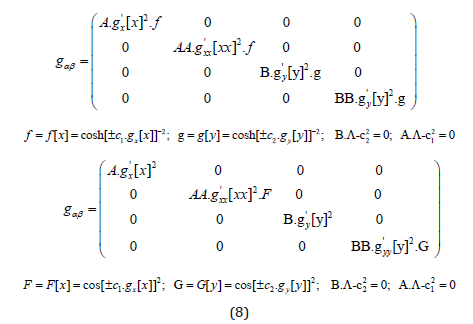
The total metric shall be given then either as:
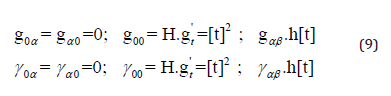
or (careful with the constant H and the function H[t]):

With the help of the results of [43,44] we can solve the
subsequent Einstein-Field-Equations and find:
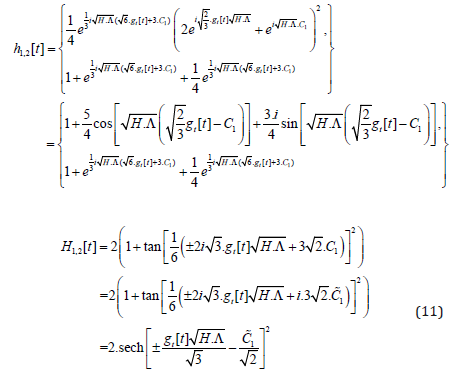
While in the case of H[t] and an assumed gt[t]=t we obtain a
bell shaped function, meaning that the whole system appears and
disappears after a while (it only virtually exists), we now have a
stable oscillation in the case h[t] (Figure 1).
Things change dramatically in the case Λ=0, where the Einstein-
Field-Equations require the following rather different solutions for
the cycle-time functions h[t] and H[t], reading:
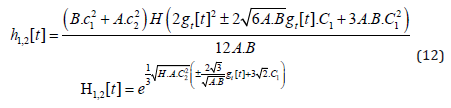
In addition, we have the functions f, g, F and G as before plus the
boundary condition for the constants A, B and ci as follows (Figure
2):

This time the approach gt[t]=t does not lead to oscillations for
h[t] but still we can achieve time-periodic behavior via H[t] and
g
t[t]=i*t.
Using the results from above it is easy to construct the metrics
for higher bit-systems. For instance, the total metric for an 8-bit
case would have to contain 17 dimensions and could generally be
given as:

We have the two options for pairwise coupling (entanglement),
namely:

By the structure given in (14), it can easily be seen how the
extension to any number of bits can be achieved.
Thin film technology has allowed to create so-called quantum
dot structures, sporting typical quantum properties in an applicable,
which is to say steerable manner. As it is widely assumed that the
realization of a Quantum Computer is achievable by using the
quantum property spin, we require an Einstein-based, which is to
say, metric understanding of this property.
Starting with the following metric:
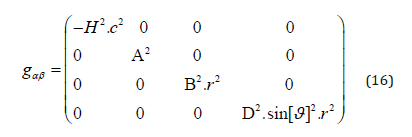
and demanding a vanishing cosmological constant

we have shown in [45] that the resulting Ricci scalar R* of the
transformed metric using recipe (2) and the corresponding 4D
wrapper-function F [f=f [t, r, ϑ, φ]] =(C
1+f) ² reads:

Now we substitute as follows in (19):

Applying the separation approach f[t,r,ϑ,φ]=g[t]*h[r]*Y[ϑ]*Z[φ]
with the previously used separation parameters C
t and m (compare
for the sub-section ”Flat Space Situation” in [33]) in (19) gives us
the following immediate solution for g[t] and Z[φ]:
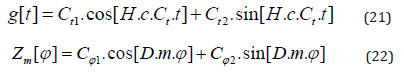
The two remaining differential equations can be constructed as
follows:
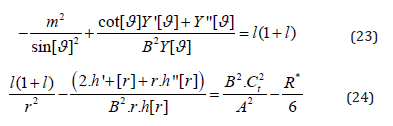
While the first equation of the two gives the usual solution with
the Legendre polynomials, as also known from the Schrödinger
hydrogen solution [46] only in slightly generalized manner (watch
the asymmetry-parameter B):
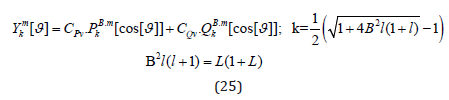
we have a rather non-Schrödinger-like solution with respect to
the radius coordinate in the case of R*=0:

Regarding the angular functions within f […] we might assume
that, just as known from the Schrödinger hydrogen problem, we
have the conditions L= 0,1,2, 3, … and -L ≤ B*m ≤ L. However, it
was shown in [33,45] that we also have options for singularity free
solutions with L= {1/2,3/2,5/2, …}. We realize that our solution
has no main quantum number as the classical Schrödinger solution
does. However, it was shown that such a number appears with the
introduction of a potential V[r]~1/r (the classical Schrödinger
way), the breaking of symmetry ([33] or appendix of [45]) or the
assumption of a boundary in r (e.g. [33]). As we are here only
interested in the half-spin solutions, we simply apply the classical
Schrödinger way and start with an approach for R* as follows:
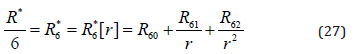
Setting this into the last line of (20) gives:
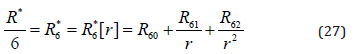
Now the classical Schrödinger wave function [46]:

directly solves our Quantum Gravity equation (28) for static
cases where f does not depend on t.
The constant a
0 is denoting the Bohr radius with (me=electron
rest mass, ε
0=permittivity of free space, Qe=elementary charge):

The functions P, L and Y denote the associated Legendre
function, the Laguerre polynomials and the spherical harmonics,
respectively.
The extension to time-dependent f according to (28) is simple.
However, in order to avoid too many parameters and work out
the connection to the classical Schrödinger evaluation, we set
H=A=B=D=1. By using the results from above we can reproduce the
Schrödinger hydrogen problem from (28) being changed to:

Thereby we have introduced the term
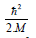
(reduced Planck
constant squared
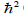
divided by mass (M) in order to truly mirror
the classical Schrödinger equation. Multiplying with M would give
us:
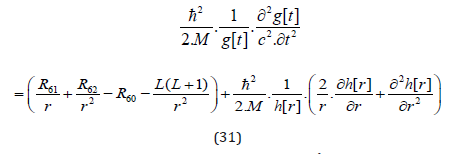
and connects the mass M with the curvature terms R6 and the
momentum.
Comparison of (31) with the Schrödinger derivation (e.g. [46],
pp. 155) and if R
60 is a constant gives us:
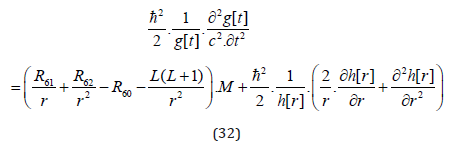
Using the result for g[t] from above, which is to say:
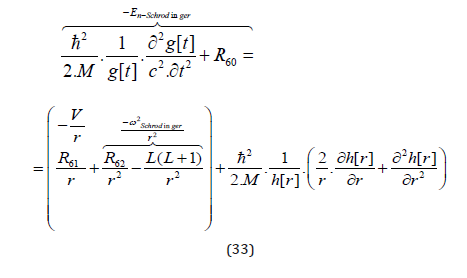
we can now rewrite (33) as follows:
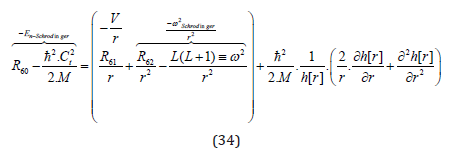
and thus, get the classical radial Schrödinger solution for the
radial part of f […]:

Regarding the conditions for the quantum numbers n, l and m,
we not only have the usual:
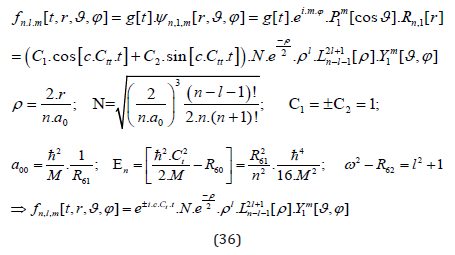
but also found suitable solutions for the half-spin forms as
discussed above and derived in the appendix. The corresponding
main quantum numbers for half-spin l-numbers with l=1/2, 3/2, …
are simply (just as before with the integers) n=l+1=3/2,5/2,7/2, l….
It should explicitly be noted, however, that the usual spherical
harmonics are inapplicable in cases of half-spin. For {n, l, m} =
{1/2, 3/2, 5/2, 7/2, …] the wave function (36) has to be adapted
as follows:
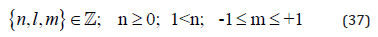
Thereby it was elaborated in [45] that in fact the sin- and the
cos-functions seem to make the Pauli exclusion (s. [47]) and not the
“+” and “-“ of the m. However, in order to have the usual Fermionic
statistic we can simply define as follows:
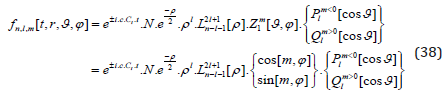
As discussed above and derived in [33] plus the appendix in
[45], the resolution of the degeneration with respect to half-spin
requires a break of the symmetry, which we achieved by introducing
elliptical geometry instead of the spherical one.
Assuming an omnipresent constant curvature R60 does not
seem to make much sense in our current system and thus, we
should set R60=0. Doing the same with the curvature parameter
R62 just gives us the usual condition for the spherical harmonics,
namely ω
2 = L(1+ L) = l
2 +1.
Thus, we result in:

This metrically derived “hydrogen atom”, which also has
half-spin solutions, was intensively discussed in [45]. Here
we concentrate on l=1/2 situations, which – so the immediate
association when illustrating the corresponding spatial distortion –
not only directly explain the Pauli exclusion principle but also allow
for a structural understanding of the half-spin solutions.
Only in order to keep things familiar and potentially compare
with the classical integer hydrogen states (40), we keep the
normalization and the scale of the Bohr radius a0. One might
perhaps call the resulting objects “half-spin hydrogen atoms”. With
only one exception (Figure 5) we start with the general setting of
m>0 ( → Z[φ]=cos[m*φ]). It can easily be seen with the simplest
half-spin states (n=3/3, l=1/2 and |m|=1/2) as presented in Figures
3&4, that the gross of deformed space-time is to be found on the
right hand side of the x=0-plane. Now choosing n=3/3, l=1/2 and
m=-1/2 and applying the statistic rule defined in (39), leading to
Z[φ]=sin[m*φ], gives us a concentration of deformed space-time
on the other side of the x=0-plane (Figure 5). It appears intuitive
to assume that the combination of objects with deformation
maxima on the left and right (anti-parallel spin) is easier than
the combination of objects having the maximum deformations on
the same sides of the x=0-plane (parallel spin combination). We
might see this as the geometric manifestation of the Pauli exclusion
principle [47].
None.
No conflict of interest.





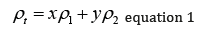
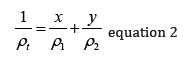

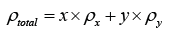

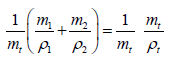
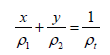


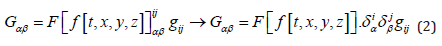
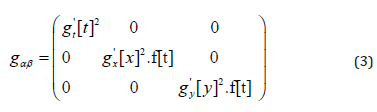

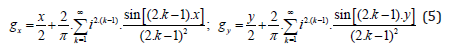
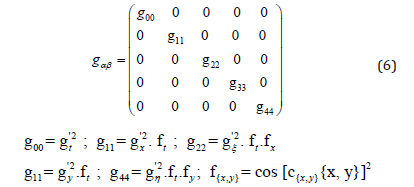
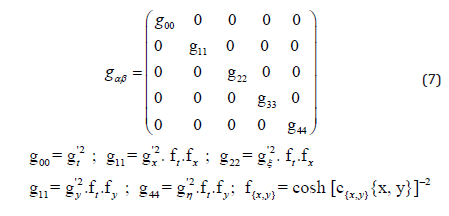
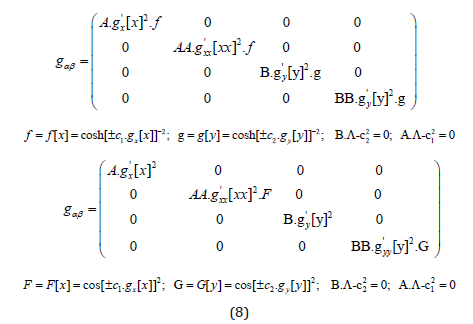
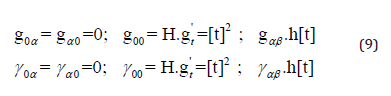

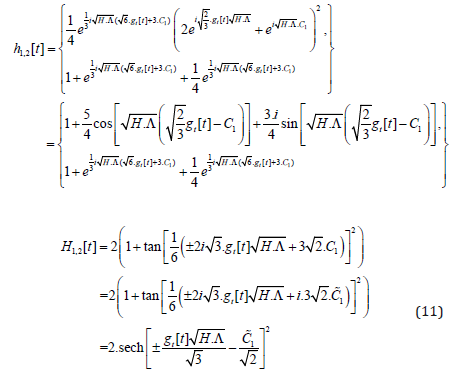
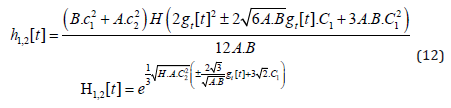



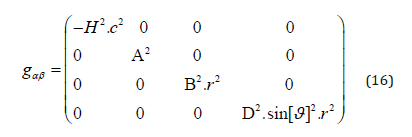



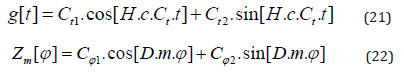
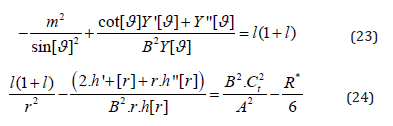
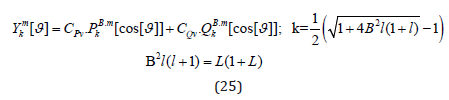

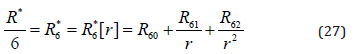



 (reduced Planck
constant squared
(reduced Planck
constant squared 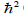 divided by mass (M) in order to truly mirror
the classical Schrödinger equation. Multiplying with M would give
us:
divided by mass (M) in order to truly mirror
the classical Schrödinger equation. Multiplying with M would give
us: