Authored by Ahmad Aijaz*
Abstract
The aim of this paper is to suggest a new lifetime distribution named as “Sauleh distribution”. Its various statistical and mathematical properties has been discussed including the shapes of pdf and cdf , moments, moment generating function, mean deviations , Bonferroni and Lorenz curves, order statistics and reliability analysis. The parameter of the distribution is estimated by using the famous method maximum likelihood estimation.
Keywords: Moments; Mean Deviation; Maximum Likelihood Estimation; Reliability; Order Statistics.
Introduction
Lindley [1] introduces one parameter distribution in the context of Bayesian statistics, as a counter example of fiducial statistics. In recent years, researchers have done lot of work on Lindley distribution and they have proposed many distributions of one parameter as well as of two parameters for modeling life time data. From biomedical and engineering point of view, some of these data provide good results and considered as life time distribution.
Ghitney [2] provided a comprehensive account of statistical properties of Lindley distribution and proved that it performs better than exponential distribution in many ways. They have also shown that the shape of the harzad rate function of the Lindley distribution is an increasing function and the mean residual life function is a decreasing function of the random variable. Recently Shanker [3] introduced a life time distribution named Akash distribution, which can be seen as modification of one parameter Lindley distribution.it has been applied on biological data and mentioned the superiority over exponential and Lindley distribution. Shanker [4-6] study various lifetime data from biomedical science and en gineering prospect and derived several distributions one of them is named as Ishita. K.k.shukla [7] proposed a new life time distribution named as Pranav distribution and studied its various structural properties. There are various situations where these life time distributions are not providing better fit either from theoretical or applied point of view.
Therefore, there is scope for searching new lifetime distribution which is more flexible than Lindley, Exponential, Ishita, Shanker, Pranav and Akash distribution, it has been described as.
One parameter distribution having parameter is defined by its pdf.
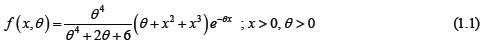
The pdf would call “Sauhleh Distribution” which is a mixture of two – distributions, Exponential distribution having scale parameter and gamma distribution having shape parameter 4 and scale parameter with their mixing proportion
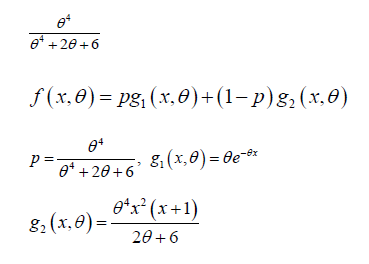
Where
The corresponding cumulative distribution function (cdf) of (1) is given as
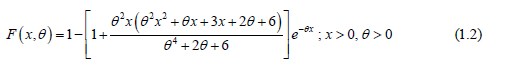
To read more about this article......Open access Journal of Biostatistics & Biometric Applications
Please follow the URL to access more information about this article
To know more about our Journals...Iris Publishers





No comments:
Post a Comment